Putting it all together!
- Select the appropriate rotation type to rebalance a BST after an insertion/removal operation is performed.
This is called a (single) right rotation:
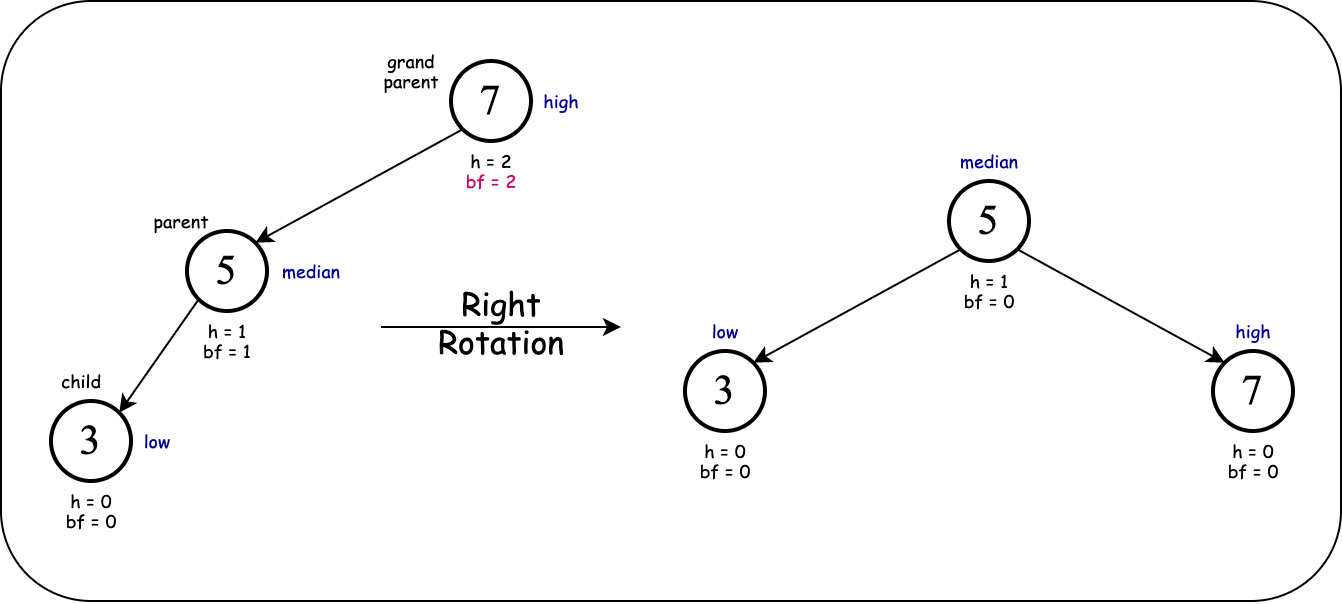
Cause of imbalance: left child's left subtree.
This is called a (single) left rotation:
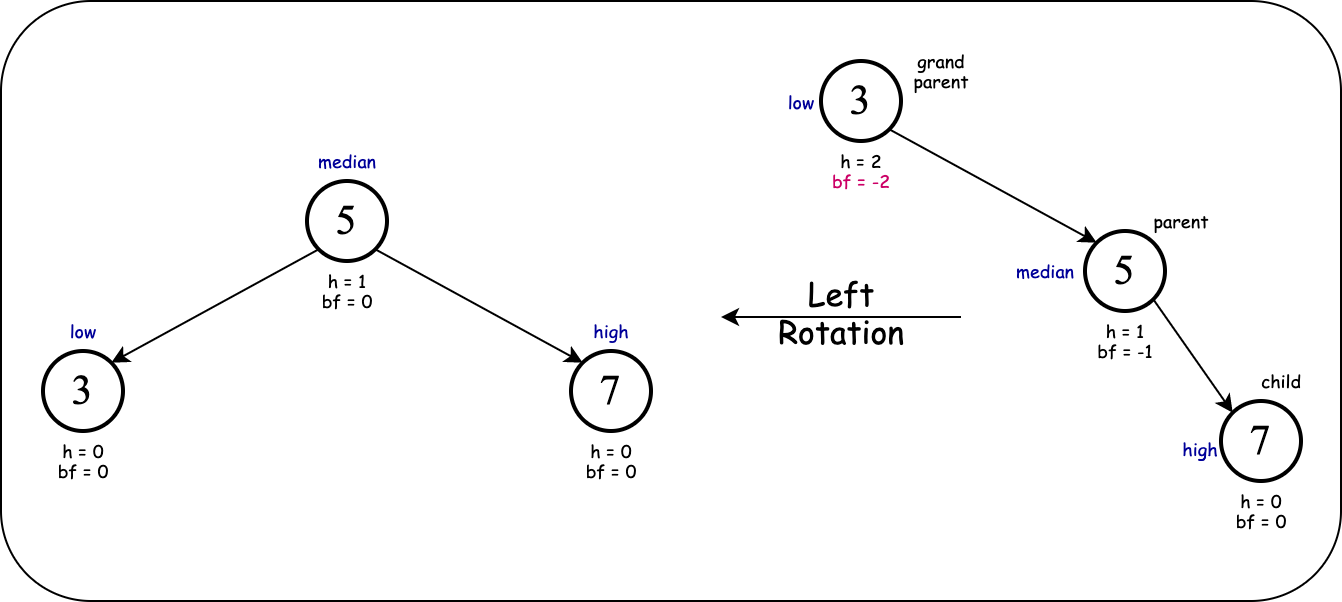
Cause of imbalance: right child's right subtree.
This is called a (double) right-left rotation:
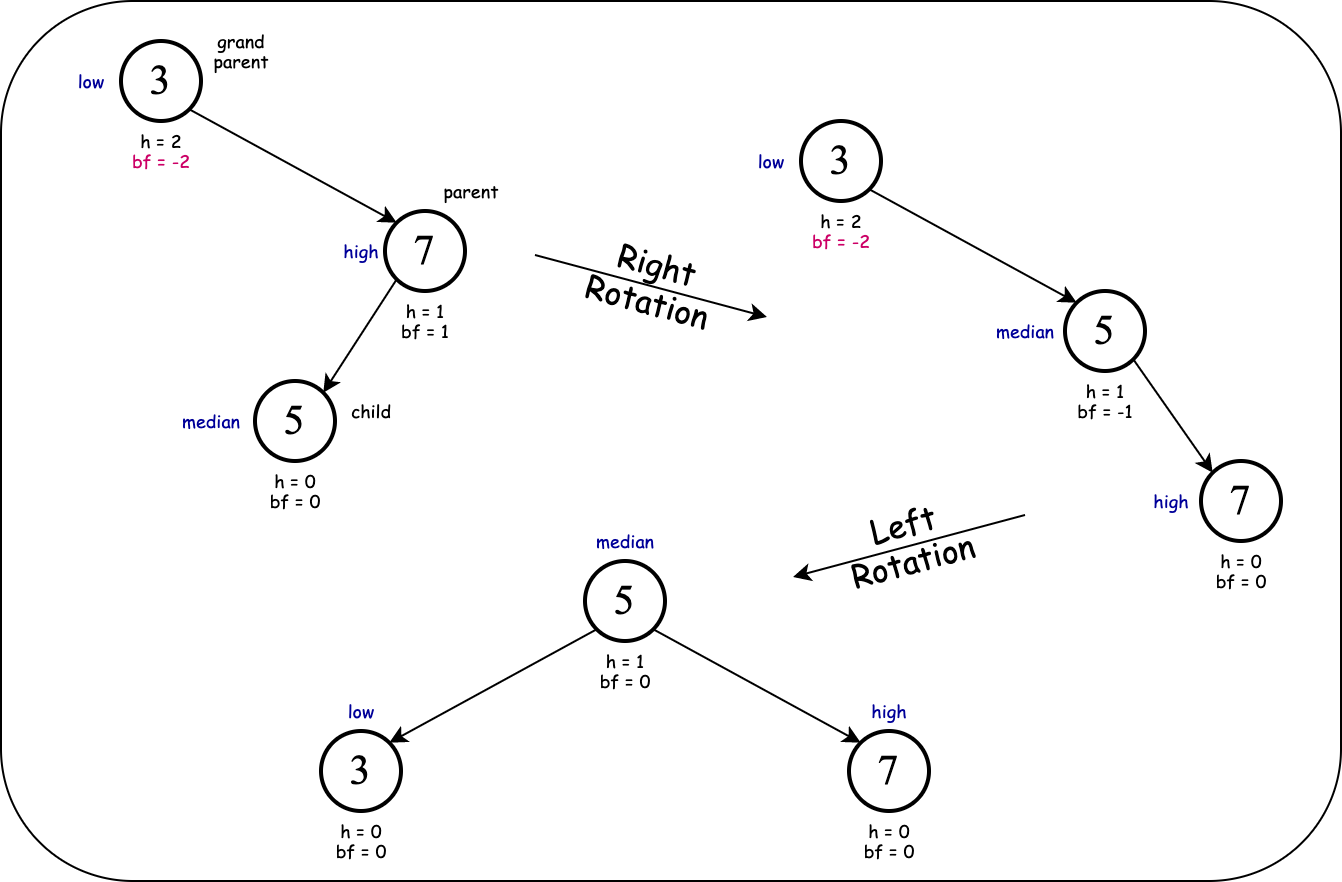
Cause of imbalance: right child's left subtree.
This is called a (double) left-right rotation:
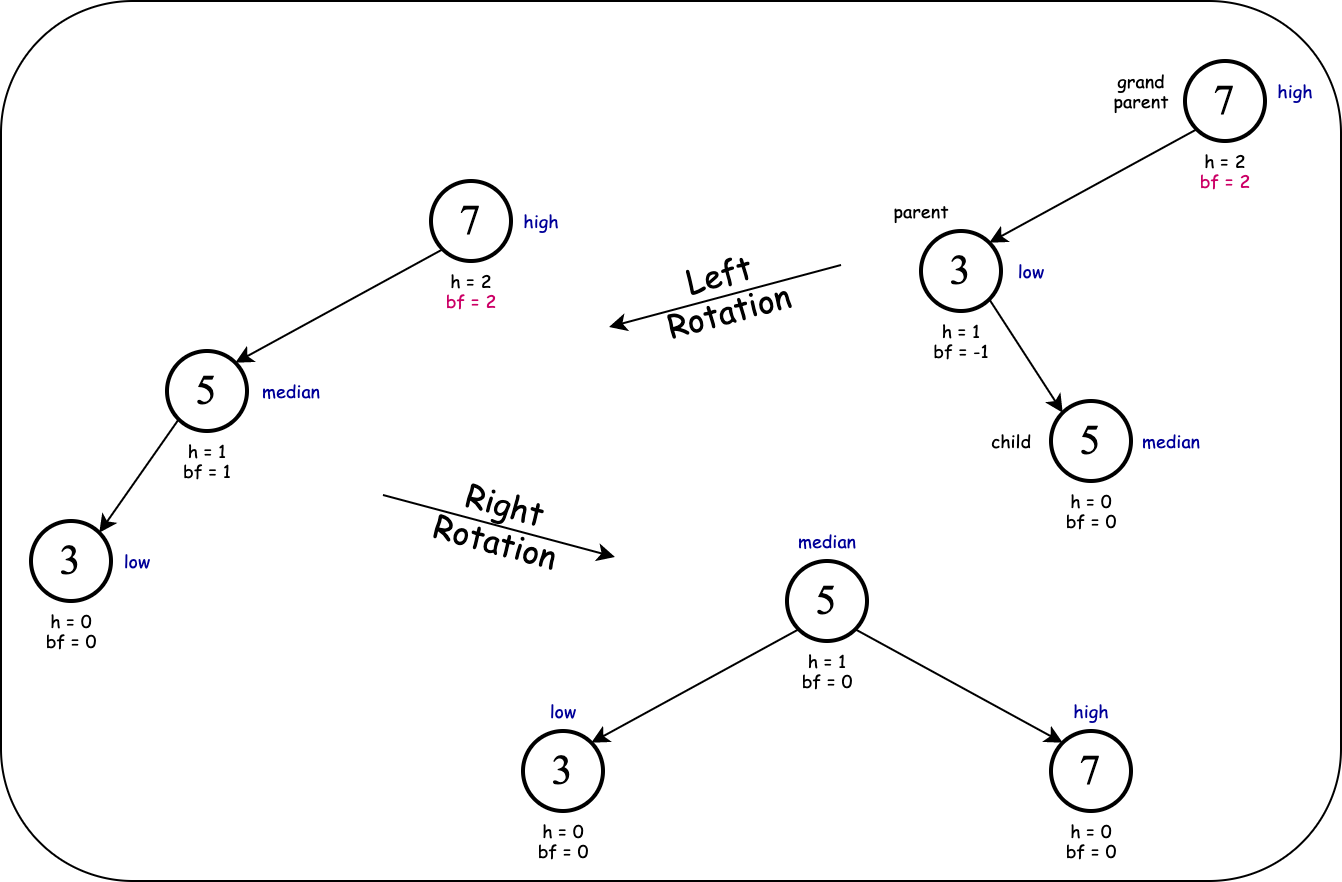
Cause of imbalance: left child's right subtree.
Exercise Complete the following table which assists in determining type of rotation.
| Imbalance Node | Child's bf = -1 (right heavy) | Child's bf = 1 (left heavy) |
|---|---|---|
bf = -2 (right heavy) | ||
bf = 2 (left heavy) |
Solution
| Imbalance Node | Child's bf = -1 (right heavy) | Child's bf = 1 (left heavy) |
|---|---|---|
bf = -2 (right heavy) | Left | Right-Left |
bf = 2 (left heavy) | Left-Right | Right |
Caution The table above does not account for an edge case where the child's balance factor is $0$.
Schematic representation of tree rotations
Resources
- Wikipedia's entry on AVL Tree: Rebalancing.
- Wikipedia's entry on Tree Rotation.